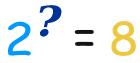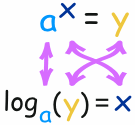Algebra
A Puzzle
What is the missing number?
| − | 2 | = | 4 |
OK, the answer is 6, right? Because 6 − 2 = 4. Easy stuff.
Well, in Algebra we don't use blank boxes, we use a letter (usually an x or y, but any letter is fine). So we write:
| x | − | 2 | = | 4 |
It is really that simple. The letter (in this case an x) just means "we don't know this yet", and is often called the unknown or the variable.
And when we solve it we write:
| x | = | 6 |
Why Use a Letter?
| Because: | |
| it is easier to write "x" than drawing empty boxes (and easier to say "x" than "the empty box"). | |
| if there are several empty boxes (several "unknowns") we can use a different letter for each one. |
So x is simply better than having an empty box. We aren't trying to make words with it!
And it doesn't have to be x, it could be y or w ... or any letter or symbol we like.
How to Solve
Algebra is just like a puzzle where we start with something like "x − 2 = 4" and we want to end up with something like "x = 6".
But instead of saying "obviously x=6", use this neat step-by-step approach:
- Work out what to remove to get "x = ..."
- Remove it by doing the opposite (adding is the opposite of subtracting)
- Do that to both sides
Here is an example:
| We want to remove the "-2" | To remove it, do the opposite, in this case add 2: | Do it to both sides: | Which is ... | Solved! |
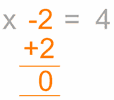 | 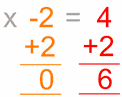 |
Why did we add 2 to both sides?
To "keep the balance"...
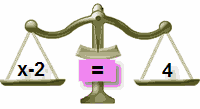 |
| In Balance |
| Add 2 to Left Side |
 |
| Out of Balance! |
| Add 2 to Right Side Also |
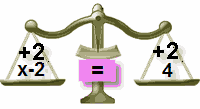 |
| In Balance Again |
Just remember this:
| To keep the balance, what we do to one side of the "=" we should also do to the other side! |
See this in action at the Algebra Balance Animation.
What is an Equation
An equation says that two things are equal. It will have an equals sign "=" like this:
| x | + | 2 | = | 6 |
That equation says: what is on the left (x + 2) is equal to what is on the right (6)
So an equation is like a statement "this equals that"
Parts of an Equation
So people can talk about equations, there are names for different parts (better than saying "that thingy there"!)
Here we have an equation that says 4x − 7 equals 5, and all its parts:
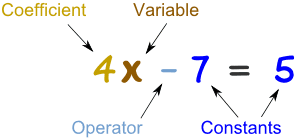
A Variable is a symbol for a number we don't know yet. It is usually a letter like x or y.
A number on its own is called a Constant.
A Coefficient is a number used to multiply a variable (4x means 4 times x, so 4 is a coefficient)
Sometimes a letter stands in for the number:
An Operator is a symbol (such as +, ×, etc) that shows an operation (ie we want to do something with the values).
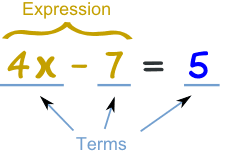
A Term is either a single number or a variable, or numbers and variables multiplied together.
An Expression is a group of terms (the terms are separated by + or − signs)
So, now we can say things like "that expression has only two terms", or "the second term is a constant", or even "are you sure the coefficient is really 4?"
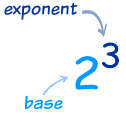
Exponents
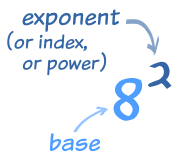 |
The exponent (such as the 2 in x2) says how many times to use the value in a multiplication.
|
Exponents make it easier to write and use many multiplications
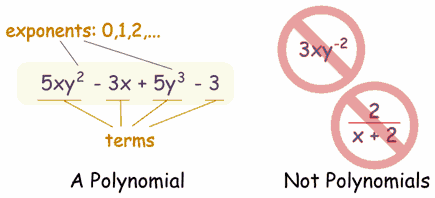
Polynomial
A polynomial can have constants, variables and the exponents 0,1,2,3,...
But it never has division by a variable.
Monomial, Binomial, Trinomial
There are special names for polynomials with 1, 2 or 3 terms:
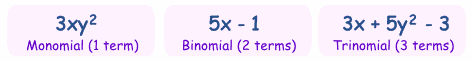
Like Terms
Like Terms are terms whose variables (and their exponents such as the 2 in x2) are the same.
In other words, terms that are "like" each other. (Note: the coefficients can be different)